73. Set Matrix Zeroes

置顶

Created At : 2021-04-07 23:59

Count:1.2k Views 👀 :

Given an m x n integer matrix matrix, if an element is 0, set its entire row and column to 0‘s.

You must do it in place.

Example 1:

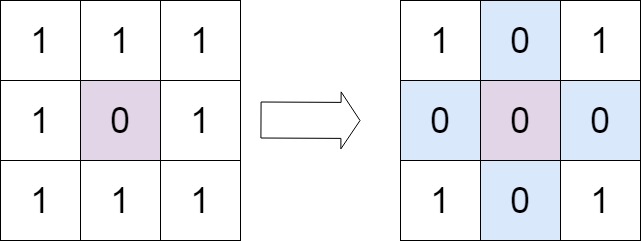
Input: matrix = [[1,1,1],[1,0,1],[1,1,1]]
Output: [[1,0,1],[0,0,0],[1,0,1]]

Example 2:

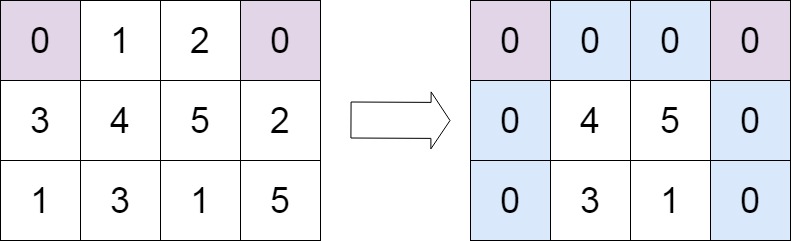
Input: matrix = [[0,1,2,0],[3,4,5,2],[1,3,1,5]]
Output: [[0,0,0,0],[0,4,5,0],[0,3,1,0]]

Constraints:

m == matrix.length
n == matrix[0].length
1 <= m, n <= 200
-2^31 <= matrix[i][j] <= 2^31 - 1

Follow up:

A straightforward solution using O(mn) space is probably a bad idea.
A simple improvement uses O(m + n) space, but still not the best solution.
Could you devise a constant space solution?

据说这题是 CareerCup 上的原题，也不算难，虽然博主也是看了网上的解法照着写的，但是下次遇到绝对想的起来。这道题中说的空间复杂度为 O(mn) 的解法自不用多说，直接新建一个和 matrix 等大小的矩阵，然后一行一行的扫，只要有0，就将新建的矩阵的对应行全赋0，行扫完再扫列，然后把更新完的矩阵赋给 matrix 即可，这个算法的空间复杂度太高。将其优化到 O(m+n) 的方法是，用一个长度为m的一维数组记录各行中是否有0，用一个长度为n的一维数组记录各列中是否有0，最后直接更新 matrix 数组即可。这道题的要求是用 O(1) 的空间，那么就不能新建数组，就用原数组的第一行第一列来记录各行各列是否有0.

- 先扫描第一行第一列，如果有0，则将各自的 flag 设置为 true
- 然后扫描除去第一行第一列的整个数组，如果有0，则将对应的第一行和第一列的数字赋0
- 再次遍历除去第一行第一列的整个数组，如果对应的第一行和第一列的数字有一个为0，则将当前值赋0
- 最后根据第一行第一列的 flag 来更新第一行第一列

解法一：

class Solution {
public:
    void setZeroes(vector<vector<int>>& matrix) {
        int m = matrix.size(), n = matrix[0].size();
        bool rowZero = false, colZero = false;
        for (int i = 0; i < m; ++i) {
            if (matrix[i][0] == 0) colZero = true;
        }
        for (int i = 0; i < n; ++i) {
            if (matrix[0][i] == 0) rowZero = true;
        } 
        for (int i = 1; i < m; ++i) {
            for (int j = 1; j < n; ++j) {
                if (matrix[i][j] == 0) {
                    matrix[0][j] = 0;
                    matrix[i][0] = 0;
                }
            }
        }
        for (int i = 1; i < m; ++i) {
            for (int j = 1; j < n; ++j) {
                if (matrix[0][j] == 0 || matrix[i][0] == 0) {
                    matrix[i][j] = 0;
                }
            }
        }
        if (rowZero) {
            for (int i = 0; i < n; ++i) matrix[0][i] = 0;
        }
        if (colZero) {
            for (int i = 0; i < m; ++i) matrix[i][0] = 0;
        }
    }
};

我们可以稍微优化一下上面的解法，少用几个 for 循环，这里只用一个变量 colZero 来表示首列是否有0存在即可，首行不用单独去记录，因为其是否为0会在 matrix[0][0] 中记录。这里只需要两个嵌套 for 循环就可以了，第一个嵌套 for 循环的i是从0开始遍历的，若首列有0，则将 colZero 标记为 true。内层 for 循环的j是从1开始遍历的，若 matrix[i][j] 为0了，则将 matrix[0][j] 和 matrix[i][0] 都赋值为0。然后开始第二个嵌套 for 循环，外层的 for 循环的i是从 m-1 往前遍历到0，内层的 for 循环的j是从 n-1 往前遍历到1，若 matrix[0][j] 和 matrix[i][0] 中任意一个为0了，则将 matrix[i][j] 赋值为0，同时在外层 for 循环的遍历过程中，判断若 colZero 为 true，则将 matrix[i][0] 赋值为0，这就保证了首列被更新了，参见代码如下：

解法二：

class Solution {
public:
    void setZeroes(vector<vector<int>>& matrix) {
        int m = matrix.size(), n = matrix[0].size();
        bool colZero = false;
        for (int i = 0; i < m; ++i) {
            if (matrix[i][0] == 0) colZero = true;
            for (int j = 1; j < n; ++j) {
                if (matrix[i][j] == 0) {
                    matrix[0][j] = 0;
                    matrix[i][0] = 0;
                }
            }
        }
        for (int i = m - 1; i >= 0; --i) {
            for (int j = n - 1; j >= 1; --j) {
                if (matrix[0][j] == 0 || matrix[i][0] == 0) {
                    matrix[i][j] = 0;
                }
            }
            if (colZero) matrix[i][0] = 0;
        }
    }
};

Github 同步地址：

https://github.com/grandyang/leetcode/issues/73

类似题目：

Game of Life

Number of Laser Beams in a Bank

Minimum Operations to Remove Adjacent Ones in Matrix

Remove All Ones With Row and Column Flips II

参考资料：

https://leetcode.com/problems/set-matrix-zeroes/

https://leetcode.com/problems/set-matrix-zeroes/solutions/26014/any-shorter-o-1-space-solution/

LeetCode All in One 题目讲解汇总(持续更新中…)

（欢迎加入博主的知识星球，博主将及时答疑解惑，并分享刷题经验与总结，快快加入吧～）